|
Mathematical Thinking in Children:
Numbers and operations
Arthur J. Baroody and Amanda R. Johnson
University of Illinois at Urbana-Champaign
Kelly S. Mix
Michigan State University
3/22/06
Paper presented at the International Conference on "Logical Mathematical Thinking in Early Childhood Education,"
sponsored by World Association of Early Childhood Educators, Madrid, Spain, April 2006.
Preparation of this paper was supported, in part, by a grant from the National Science Foundation (BCS-0111829)
and the Spencer Foundation (200400033). The opinions expressed are solely those of the authors and do not necessarily reflect the
position, policy, or endorsement of the National Science Foundation or the Spencer Foundation.
Changing Views
Over the course of the twentieth century, psychologists came to dramatically different conclusions about-
1. the nature of young children's mathematical competence (i.e., what informal mathematical strengths
preschoolers possessed) and
2. its basis (e.g., what role language plays in concept development.
>1. Changes in the Conventional Wisdom Regarding the Nature
of Preschoolers' Informal Knowledge
Like a pendulum, the conventional wisdom about young children's number and arithmetic competence has
swung from extremely pessimistic to extremely optimistic and then back toward the middle.
a. Early Pessimistic Views. For most of the century, psychologists held a pessimistic view and
focused on what children can't do.
. William James (1890):
an infant's perception of the world =
a "great, blooming, buzzing confusion."
. Edward L. Thorndike (1922):
young children are so mathematically inept that "little is gained by [doing] arithmetic before grade 2,
though there are many arithmetic facts that can [be memorized by rote] in grade 1" (p. 198).
. Jean Piaget (1965):
Piaget's relatively pessimistic views about young children's abilities and capacities also had
the effect of limiting expectations about what they can learn and be taught. For instance, he believed that preschoolers
are preoperational thinkers, incapable of logical and systematic thinking or constructing abstract concepts
(e.g., a true concept of number or understanding of arithmetic).
The pessimistic views of social theorists reinforced a minimalist approach to early
childhood mathematics instruction.
For example, in response to surveys of instructional practices by Juanita Copley
(University of Houston, 2004), early childhood teachers often make such comments as:
."I don't do mathematics."
."Young children shouldn't do mathematics . . . it's not appropriate."
Until recently, these responses reflected the prevailing attitude in our culture toward
teaching preschoolers mathematics. Other than perhaps have children memorize the counting sequence, the names of
written numbers such as 3 and 4, some arithmetic facts, and the names of simple shapes, parents, preschool caregivers,
publishers, and the media paid little attention to mathematics instruction.
b. The Shift to a Highly Optimistic View. In the last quarter of the 20th century,
psychologists adopted a highly optimistic view and focused on what children can do (Gelman, 1979).
For example, Wynn (1998) noted, "Findings over the past 20 years have shown infants are sensitive
to number" (p. 5). Specifically, she argued they are born with an ability to recognize and distinguish among
oneness, twoness, and threeness and can even reason about or operate on very small numbers
(e.g., recognize that one object added to another makes two and that two objects minus one is one)-all before they d
evelop verbal-based counting competencies.
Indeed, Gelman (e.g., Gelman & Meck, 1992) argued that children are innately endowed with counting
principles-principles that allow infants to nonverbally count (using nonverbal tags or representations) and toddlers to
quickly learn number words and how to use them to count collections.
c. The Recent Shift to Middle Ground Views. Some research over the last 10
years indicates that nativists such as Wynn (1992, 1998) may be too optimistic and that a more balanced view
of children's informal mathematical knowledge is needed.
If nativists are correct and children are born with a nonverbal and innate concept
of one to at least three, they should not have difficulty with non-verbal number and arithmetic
tasks that involve these "intuitive numbers.
Consider the case of 4-year-old Shytiesha (Videotape segment #1).
Task 1: "Fair Battle" (Non-Verbal Matching).
Note that Shytiesha correctly created a matching collection when the tester's collection
consisted of only one or two items.
Note that she put out four items when the tester's collection consisted of three or four items.
That is, she treated collections of three and four as equivalent.
Shytiesha's performance illustrates what we have commonly found: Young children's number
performance drops off dramatically when collections are larger than 2 items.
That is, there seems to be a serious gap between their ability
to deal with (1 or) 2 and 3 (or larger numbers). Nativists would predict that the drop should be between 3 and 4.
Task 2: "Hiding Game" (Non-Verbal Production).
Note that this task, unlike the matching task, requires a child to form a mental
representation of the tester's collection, because the tester hides his collection after showing it for 3 seconds.
Note that Shytiesha correctly re-creates the tester's collection when it consisted of only one or two items.
Note that she incorrectly labels the tester's collection of four items as "three" and then puts out four items.
Note that she again puts out four items when the tester's collection was three.
For Shytiesha, the number word "three" does not appear to have the same meaning as an adult.
She appears to use "three" to mean "many."
Task 3: "Double Hiding Game" (Mental Representation and Non-Verbal Production of Two Collections).
Note that Shytiesha now labels the collection of four as " bunch." So four items is a "bunch," "three," or many.
Note that she correctly labels and reproduces the collection of two.
Note that Shytiesha concludes, "It's (the collection of 4) is the same"
(as the collection of 3). "It's a bunch." So again, she treats three and four items as equivalent (as many).
Not only is there a drop off in performance between 2 and 3, young children's zone
of comfort is exceeded far more quickly than nativists' would predict.
Dowker (1997, 1998, 2003) identified three levels of mental arithmetic competence among
elementary school children-levels that can also be applied to number competence (Baroody, Benson, & Lai, 2003):
1. The zone of competence (familiarity and comfort) in which they
can determine exact answer by computation or recall-or, in the case of a collection, can visually recognize and
accurately specify the cardinal (total) value of the collection.
2. The zone of proximal competence (moderate familiarity and comfort)
in which they can effectively use existing knowledge to estimate the answer-or, in the case of a collection,
can reasonably approximate the cardinal value of the collection.
3. The zone of incompetence (unfamiliarity and discomfort) in which they "melt down"
(e.g., resort to a wild guess or refuse to respond).
Consider the case of 3 year-old Haley (Videotape segment #2).
"Hiding Game" (Non-Verbal Production).
Note that for that for the trials involving 3 and 4, Haley responded by simply putting out
all the items available (i.e., she melted down and did not try to produce an exact or approximate answer).
"Double Hiding Game" (Non-Verbal Production of Two Collections).
In the first trials (1 & 1 and 3 & 2), note the value of labeling a collection
with a number word ("one" and "two" serve as valuable memory aids).
With the second and third trial (3 & 2 and 2 & 4), Haley does not reproduce 3 or 4
accurately but at least responds with a good estimate.
Note that she labels 4 as "three," which seems to mean "many" to her.
"Double Hiding Game" (Non-Verbal Production of Two Collections)-continued.
Note that for the last (4 & 3) trial, Haley looks at the tester's two collections
and concludes: "It's (the collection of 3) just like that one (the collection of 4). That is, for her 3 and
4 are equivalent and are both "many."
Note that she appears to melt down by putting out nearly all the items available (7 of the 8).
Note that Haley then engages in problem solving. She apparently recognizes that
both of her mats should have "many," but that she has only 1 item left for the second mat. She then proceeds
to borrow four chips from the first mat to represent "many" on the second mat-leaving three chips ("many") on the first mat.
Consider some additional examples of melting down.
14.Two-year-old
child correctly creates a matching collection by putting out 2 dinosaurs.

15. Shown 4, the child puts out
all items available (melts down)

16. Asked to match 4 again, child
pushes dinosaurs away.

17. And then pushes his mat into the
tester's mat.

18. Tester shows 2-year-old child 2
dinosaurs.

19. Tester hides 2 dinosaurs.

20. Child puts out one.

21. Tester shows and hides 2 dinosaurs
again.

22. Child simply grabs a bunch with each hand (melts down).

23.Tester shows and then hides 4 dinosaurs.

24. Child leaves (melts down).

The research just discussed has the following important educational implication.
Instruction (and testing) should be sensitive to children's developmental level in general
and their individual zones of competence, partial competence, or incompetence in particular.In order to
ensure that external factors mesh with internal ones so as to promote meaningful learning (Dewey, 1963), preschool
teachers should be aware that what constitutes an overwhelmingly large number varies from child to child and that
this key internal factor can involve very small numbers and may be narrow (exceeded quickly).
2. Changes in the Conventional Wisdom About the Basis of Preschoolers' Informal Knowledge
of Number and Arithmetic
Like a pendulum, the conventional wisdom about the role of language in number development has
also shifted back and forth over the last 100 years or so (see Mix, Sandhofer, & Baroody, 2005, for a detailed discussion).
a. Early Counting-Based View. Dewey (1898) and Thorndike (1922) concluded that children's
initial training in mathematics should focus on counting.
b. Nonverbal Number Concept Before a Verbal-Based Concept View. For most of the 20th century,
psychologists have believed that a number concept develops independently of counting or before children acquire an
understanding of verbal numbers.
. Piaget (1965), for example, dismissed verbal and object counting as skills learned by
rote, skills that had no impact on constructing a number concept. He argued that the construction of a number concept
depended on the development and synthesis of the logical thinking abilities necessary for classifying and ordering.
. Nativists have argued that children have an innate non-verbal concept of at least the
intuitive numbers.
c. Language in the Form of the Number Words Plays a Central Role in
the Formation of Number, Counting, and Arithmetic Concepts (and probably those of geometry and other
aspects of mathematics).
. Concept of Number (Verbal Number Recognition). The use of the first few counting
words ("one," "two," "three") in conjunction with seeing examples and non-examples of each can help
children construct an increasingly reliable and accurate number concept of one, two, and three-an understanding
of oneness, twoness, and threeness. By seeing ··, DD, and oo (examples of pairs), e.g., all labeled "two,"
young children can recognize that the appearance of the items in the collections is not important (shape and color
are irrelevant to number). Seeing ·, ···, D, DDD,  , and
, and  (non-examples of pairs) labeled as
"not two" or with some number word can help them define the boundaries of the concept of "two" and more accurately
or selectively apply this word. (non-examples of pairs) labeled as
"not two" or with some number word can help them define the boundaries of the concept of "two" and more accurately
or selectively apply this word.
. Meaningful Object Counting. Verbal number recognition (VNR) can also serve as the foundation
for meaningful object counting or enumeration. Once children can reliably and meaningfully recognize and label small
collections, the use of this conceptually based VNR skill in conjunction with seeing enumeration modeled by others can
enable children to understand the why and the how of the latter. Specifically, meaningful VNR can enable children to
discern the purpose of enumeration (its another way of determining the total number of items of a collection or its cardinal
value) and the rationale for enumeration procedures (e.g., why others emphasize or repeat the last number word used in the
enumeration process because it represents the total number of items or cardinal value of the collection.
. Concept (Big Idea) of Decomposition and Composition. VNR also enables
children to see two as one and one and three as two and one or as one and one and one-as a collection
composed of units or a whole composed of individual parts (Freeman, 1912). Such experiences provide the basis
for a fundamental understanding of the "big idea" of decomposition and composition.
. Addition. A fundamental understanding of decomposition and composition
also provides the basis for constructing a basic understanding of the part-whole relations that under-gird
an informal concept of addition. For example, by adding an item to a collection of two items, a
child can literally see the original collection has been transformed into a larger collection of three.
This may provide the basis for constructing an informal change-add-to concept of addition.
The key instructional implication is that helping children develop an ability to verbally
recognize the intuitive numbers (1 to about 3) by labeling examples and non-examples is critical basis
for mathematics instruction and, indeed, should be accomplished before trying to teach children object counting.
Another key instructional implication is that teachers should focus on helping
students discover and understand big ideas-key ideas that underlie numerous concepts and procedures
across topics.
As Paulos (1991) advised, "stress a few basic principles and [leave] most of the details
to [the student]" (p. 7). If students understand the big ideas, most will be able to rediscover or
reinvent the principles, properties, and procedures central to elementary arithmetic and geometry, including the renaming procedures, the commutative and distributive principles, and area formulas. Understanding big ideas can help students understand the rationale for specific methods (e.g., procedures and formulas), adapt them to meet the challenge of new problems or tasks ("adaptive expertise"), and see how various concepts and procedures are related. This can help students see that mathematics is a system of knowledge. This in turn can make learning diverse ideas and procedures much easier.
For example, the big ideas of composition-parts can be
combined (composed), often in different ways, to form the whole-and decomposition-
whole can consist of parts and be broken apart (
decomposed) into them, often in different ways-underlies various aspects or topics of mathematics:
· Other names for a number (e.g., different parts can compose to make the
same whole, such as 1 + 7, 2 + 6, 3 + 5, and 4 + 4 = 8. and a whole can be decomposed in constitute
parts in different ways, such as 8 = 1 + 7, 2 + 6, 3 + 5, 4 + 4.)
· invention of reasoning strategies and mastery of larger addition
combinations (e.g., 7+ 8 = 7 + [7+ 1] = [7 + 7] + 1 = 14 + 1= 15 or 7+ 8 = [7 - 2] + [8 + 2] = 5 + 10 = 15)
· part-whole number relations (e.g., one of two or more parts is smaller
than its whole and conversely a whole is larger than any one of its multiple parts)
· missing-addend (part) problems (e.g., in the problem below or in the
equation 4 + ? = 6, the missing part must be smaller than the whole 6 and when added to 4 equals 6)
n Georgia had 4 dresses. Her mother bought her some more. Georgia found 6 dresses
hanging in closet. How many new dresses did Georgia's mom buy?
· invention of reasoning strategies and mastery of subtraction
combinations (e.g., for 6 - 4 = ?, the whole 6 minus the part 4 is equal to the other part, which
when added to 4 makes 6; i.e., 6 - 4 = ? is related to the missing-addend expression 4 + ? = 6 and,
because 4 + 2 = 6, the unknown part is 2)
·renaming (carrying and borrowing) procedures(e.g., for 37 + 28, a child must be
able to recognize that the sum of the ones digits 15 can be decompose into a 10 and a 5 and that this
10 must be added to the three 10s and two 10s shown in the 10s place)
·geometry (e.g., a square can be decompose into two [right, isosceles]
triangles and vice versa).
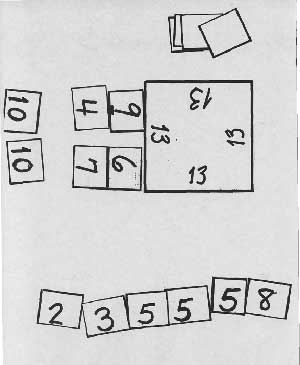
Examples of a Composition-Decomposition Activity: The Number Goal Game
Two to six children can play this game. A large center card (square) is
placed in the middle with a number such as 13 printed on it. Each player draws six small squares numbered 1
to 10 from a pile of squares all facing down. The players turn up their squares. On his or her turn, a player
may compose two or more squares to a sum equal to the number in the center.
If a player had squares 2, 3, 5, 5, 5 and 8, she could combine 5 and 8 and
3, 5, and 5 to make 13. As each solution would be worth 1 point, the player would get two points
for the round. If the player had chosen to combine 2 + 3 + 8, no other possible combinations of 13 would be
left, and the player would have scored only 1 point for the round.
An alternative way of playing (scoring) the game is to award points for both the number of parts
used to compose the target number (e.g., the play 3 + 5 + 5 and 5 + 8 would be scored as 5 points, whereas the
play 2 + 3 + 8 would be scored as 3 points).
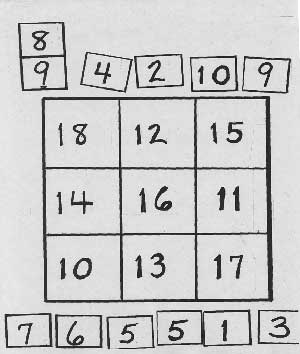
Second Example of a Composition Decomposition Activity: Number Goal-Tic Tac Toe
(or Three in a Row)
This game is similar to the Number Goal Game. Two children can play this game.
Each player draws six small squares numbered 1 to 10 from a pile of squares all facing down. The players turn
up their squares. On his or her turn, a player may compose two or more squares to create a sum equal to one
of the numbers in the
3 x 3 grid. If a player can do this, s/he places her/his marker on the sum in
the 3 x 3 grid, discards the squares used, and draws replacement squares. The goal is the same
as Tic-Tac-Toe-that is, to get three in a row.
Using Egyptian Hieroglyphics to Underscore Decomposition and Base
Ten/Place-Value Ideas
Based on Investigation 6.1
(Baroody with Coslick (1998)
| = 1  = 10
= 10  = 100
= 100
Challenge student to find how many ways the digits
1, 2, and 5 can be combined to form a three-digit numeral. Ask them if 153, 215, 251, 512, and 521
represent the same number as 125 (Whitin & Wilde, 1992). Repeat the challenge with Egyptian
hieroglyphics. Discuss the similarities and differences between our Arabic numerals and Egyptian hieroglyphics.) |